GTx: Engineering Vibration I: Introduction: Single-Degree-of-Freedom Systems
This course is an introduction to exploring the topic of engineering systems undergoing vibration.

- Duration
- 5 weeks
- Price Value
- $ 199
- Difficulty Level
- Introductory
This course is an introduction to exploring the topic of engineering systems undergoing vibration.


Welcome to "Engineering Systems in Motion: Vibrations of Engineering Systems," an exciting introductory course that delves into the fascinating world of vibration in engineering structures. This course, offered by GTx, provides a comprehensive exploration of vibration analysis, focusing on Single Degree-of-Freedom (SDOF) systems. You'll learn essential methods to predict and analyze the vibratory motion of engineering structures in response to various inputs. Whether you're an aspiring engineer or a curious learner, this course will equip you with valuable skills to understand and tackle real-world engineering challenges.
Students must have successfully completed the course "Engineering Systems in Motion: Dynamics of Particles and Bodies in Planar Two-Dimensional (2D) motion," which is available for free on Coursera. This prerequisite ensures that students have the necessary foundational knowledge to excel in this vibration-focused course.
This course is ideal for engineering students, professionals seeking to expand their knowledge in vibration analysis, and anyone interested in understanding the principles of mechanical vibrations in engineering systems. It's particularly suited for those who have a background in basic engineering dynamics and want to delve deeper into specialized vibration analysis.
The skills acquired in this course have numerous practical applications in engineering and industry. Learners will be able to:
Week 1 – Vibration System Modeling Elements
Week 2 – Vibration System Differential Equations of Motion
Week 3 – Undamped Single Degree-of-Freedom Vibration Systems
Week 4 – Damped, Single-Degree-of-Freedom Vibration Systems
Week 5 – Damped, Single-Degree-of-Freedom Vibration Systems (continued)
Each week contains multiple modules covering specific topics, from basic concepts to advanced analysis techniques. The course concludes with a comprehensive look at various types of damped vibration systems and their practical applications.
Explore more courses to enhance your cloud computing and Kubernetes skills.

Study of ordinary differential equations (e.g., solutions to separable and linear first-order equations and to higher-order linear equations with constant coefficients, systems of linear differential equations, the properties of solutions to differential equations) and linear algebra (e.g., vector spaces and solutions to algebraic linear equations, dimension, eigenvalues, and eigenvectors of a matrix), as well as the application of linear algebra to first-order systems of differential equations and the qualitative theory of nonlinear systems and phase portraits.

Learn the fundamental principles underlying fluid dynamics; including the kinematics of deformation, hydrostatics & buoyancy, inviscid flow and the application of Bernoulli’s theorems, as well as applications of control volume analysis for more complex problems of engineering interest.
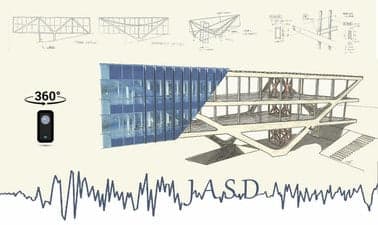
In this revised course, fundamental and modern approaches to Japanese structural design will be explained using historical overviews and Tokyo-Tech’s campus buildings as case studies. Learners will be able to interpret and apply seismic design concepts like energy-dissipating braced frames, spine frames, seismic retrofit, seismic isolation and seismic design of spatial structures. Drawing from the instructor’s past 20 years of experience in design, considerations to sustainability, practical complexities and their solutions will be presented using the campus buildings as design examples with immersive 360-degree interactive videos. Learners will also get an insight into the architect-engineer collaboration through interviews with architectural professors.
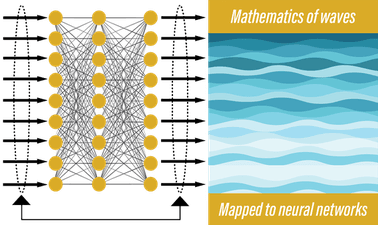
All around us, information is carried by waves of many kinds, like acoustic, electromagnetic, gravitational or probability waves. Enjoy this unifying view of all wave phenomena using neural networks to visualize the underlying mathematics.

Physicists use math all of the time in nearly everything that they work on. This course will help you understand how math is interconnected and recognize that math involves a handful of simple ideas that repeat. By the end of the course, you will be able to re-derive important formulas from basic principles or know precisely where to look them up and use them.