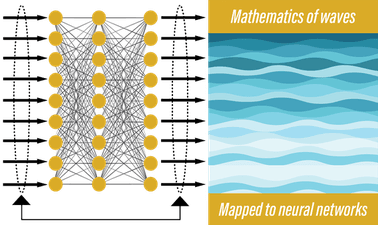
PurdueX: Mathematics of Waves: Visualized with Neural Networks
All around us, information is carried by waves of many kinds, like acoustic, electromagnetic, gravitational or probability waves. Enjoy this unifying view of all wave phenomena using neural networks to visualize the underlying mathematics.
- Duration
- 2 weeks
- Difficulty Level
- Intermediate